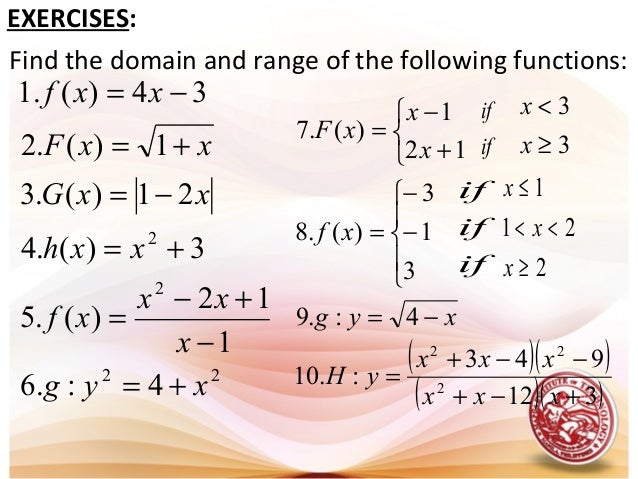
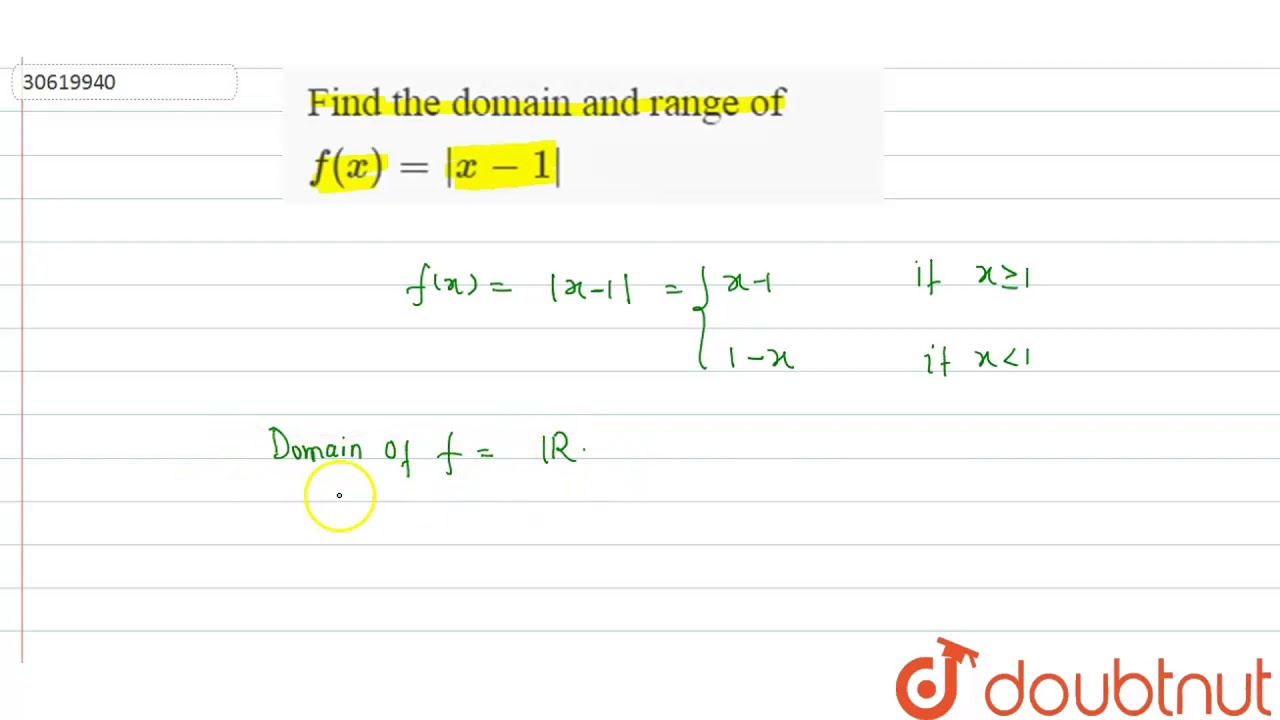
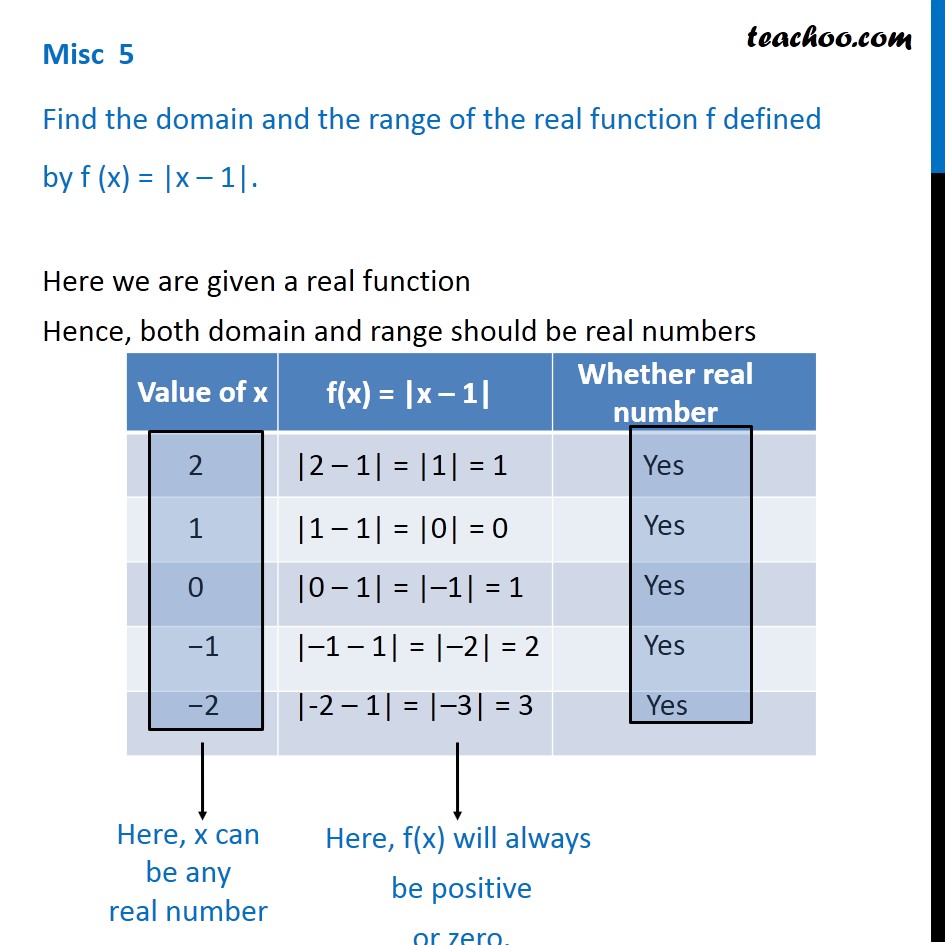
What is the domain and range of f (x) = 1/x?Find the Domain and Range f (x)= (x1)/ (x1) f (x) = x − 1 x 1 f ( x) = x 1 x 1 Set the denominator in x−1 x1 x 1 x 1 equal to 0 0 to find where the expression is undefined x1 = 0 x 1 = 0 Subtract 1 1 from both sides of the equation x = −1 x = 1 Transcript Misc 5 Find the domain and the range of the real function f defined by f (x) = x – 1 Here we are given a real function Hence, both domain and range should be real numbers Here, x can be any real number Here, f (x) will always be positive or zero Here value of domain (x) can be any real number Hence, Domain = R (All real numbers) We note that that range f (x) is 0 or positive numbers, So range cannot be negative Hence, Range

How To Find The Domain And Range Of A Function 14 Steps
F x x 1 domain and range
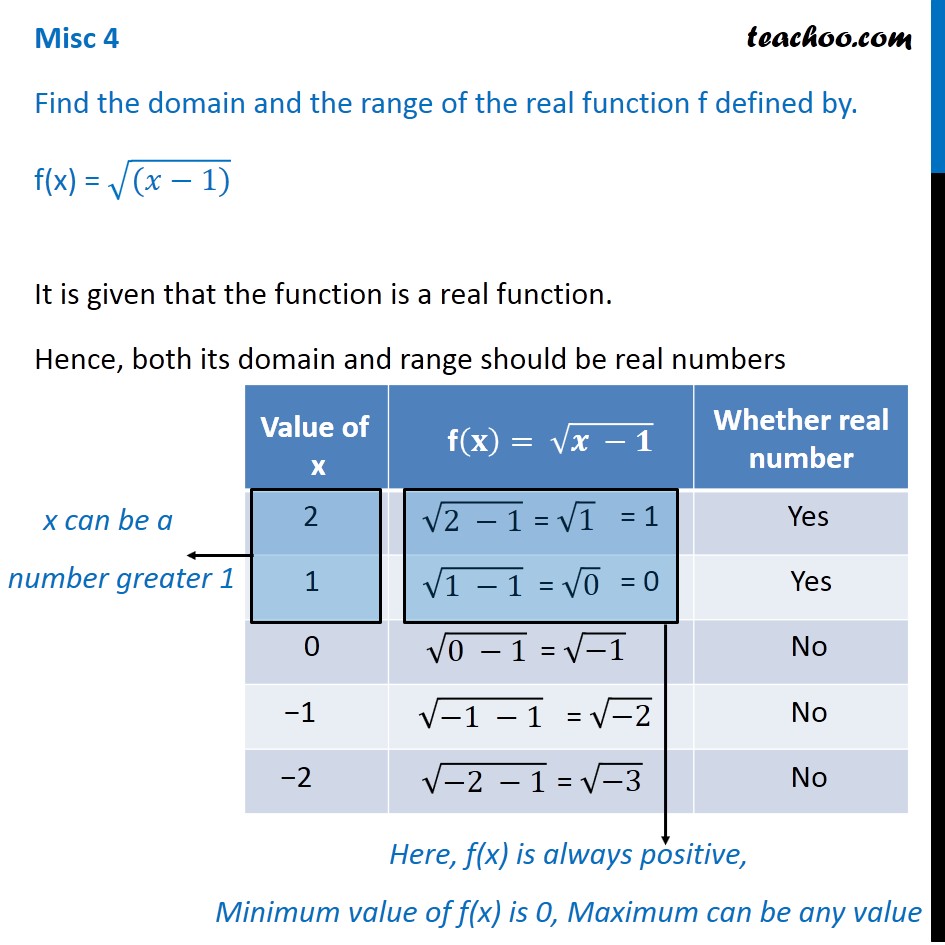
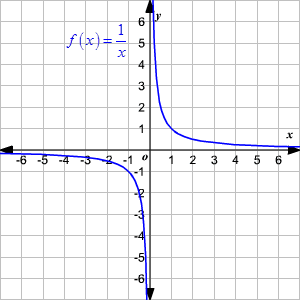
F x x 1 domain and range- Misc 4 Find the domain and the range of the real function f defined by f(x) = √((𝑥−1)) It is given that the function is a real function Hence, both its domain and range should be real numbers x can be a number greater 1 Here, f(x) is always positive, Minimum value of f(x) is 0,Find the Domain and Range f (x)=1/x f (x) = 1 x f ( x) = 1 x Set the denominator in 1 x 1 x equal to 0 0 to find where the expression is undefined x = 0 x = 0 The domain is all values of x x that make the expression defined Interval Notation




What Are The Domain And Range Of F X 1 5 X Brainly Com
2x 1 = 0 2x = 1 x = 1 / 2 Therefore , Domain = R { 1/2 } All real numbers are used as range here For getting solve the denominator of the given fraction and reduce the value of x from the real number "R" Hope it helps you dudeDomain and Range of Exponential and Logarithmic Functions Recall that the domain of a function is the set of input or x values for which the function is defined, while the range is the set of all the output or y values that the function takes A simple exponential function like f(x) = 2x has as its domain the whole real lineFor example, a function f (x) f ( x) that is defined for real values x x in R R has domain R R, and is sometimes said to be "a function over the reals" The set of values to which D D is sent by the function is called the range Informally, if a function is defined on some set, then we call that set the domain
The given real function is f (x) = x − 1 It can be seen that x − 1 is defined for (x − 1) ≥ 0 ie, f (x) = (x − 1) is defined for x ≥ 1 Therefore the domain of f is the set of all real numbers greater than or equal to 1 ie, the domain of f = 1, ∞)To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW Find domain and range of `f(x)=x/(1x^2)`The Domain and Range of a Function The domain of a function is the set of values for the variable in which the function is defined or real On the
We can see the function a define for all values of x Domain of f(x) = R, where R is the set of all real number When you put any value of x from domain you'll get either '0′ or any positive value for f(x) So, Range of f(x) = 0, ∞) Graphical Method Simply draw the graph and you can see domain & range in graph itselfRange {yly>0} domain {x\x> 1/6);All real y ≥ 0 Example a State the domain and range of y = √ x4 b Sketch



L1 Functions Domain Amp Range



Find The Domain And Range Of The Function F X Chegg Com
To find the domain, let f(x)=1/log(2x) is not defined for 1 2xFunction should remain real so math√(x^2–1)/mathshould not be equal to zero so math x^2–1 \neq 0/math mathx^2=1/math Or mathx=\pm 1/math And f (x) = 1/√x−5 Now for real value of x5≠0 and x5>0 ⇒ x≠5 and x>5 Hence the domain of f = (5, ∞) And the range of a function consists of all the second elements of all the ordered pairs, ie, f(x), so we have to find the values of f(x) to get the required range Now we know for this function x5>0 taking square root on both



Find Domains And Ranges Of The Toolkit Functions College Algebra




Find The Domain And Range Of F X Root Of X 1 Brainly In
Range {yly> 2} domain {x x g According to the United States Postal Service, First Class Mail International (letters) takes 6 to days to deliver to their destination In order to test this, Greg, in Chicago, sent oneFind the domain and range of the function `f (x)= (1)/sqrt (x5)` Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't begin shortly, try restarting your device UpThe domain and range are the intervals in which the function is defined in the x and y axes Answer and Explanation 1 Become a Studycom member to unlock this answer!



What Is The Domain And Range Of 1 X 3 Quora



View Question What Is The Domain And Range For The Function F X 1 9 X 2
Find Domain and Range of real functions (1) `f(x)=(x2)/(3x)` (2)`f(x)=1/sqrt(x5)` (3) `f(x)=x/(1x^2)` 1 f(x)= x 1 Find the domain, range, and intercepts of the function Then find the minimum and maximum values on the interval 0, 3I'll be breaking down this question in three parts, 1 When x is positive 2 When x is negative 3 When x is zero In the first case, if x is positive, And since the modulus of a positive number is the number itself, our function becomes, f(x) = x/




If The Function F X Has A Domain Of 2 X 8 And A Range Of 4 Y 6 And The Function G X Is Defined By The Formula G X 5f 2x Then What Are
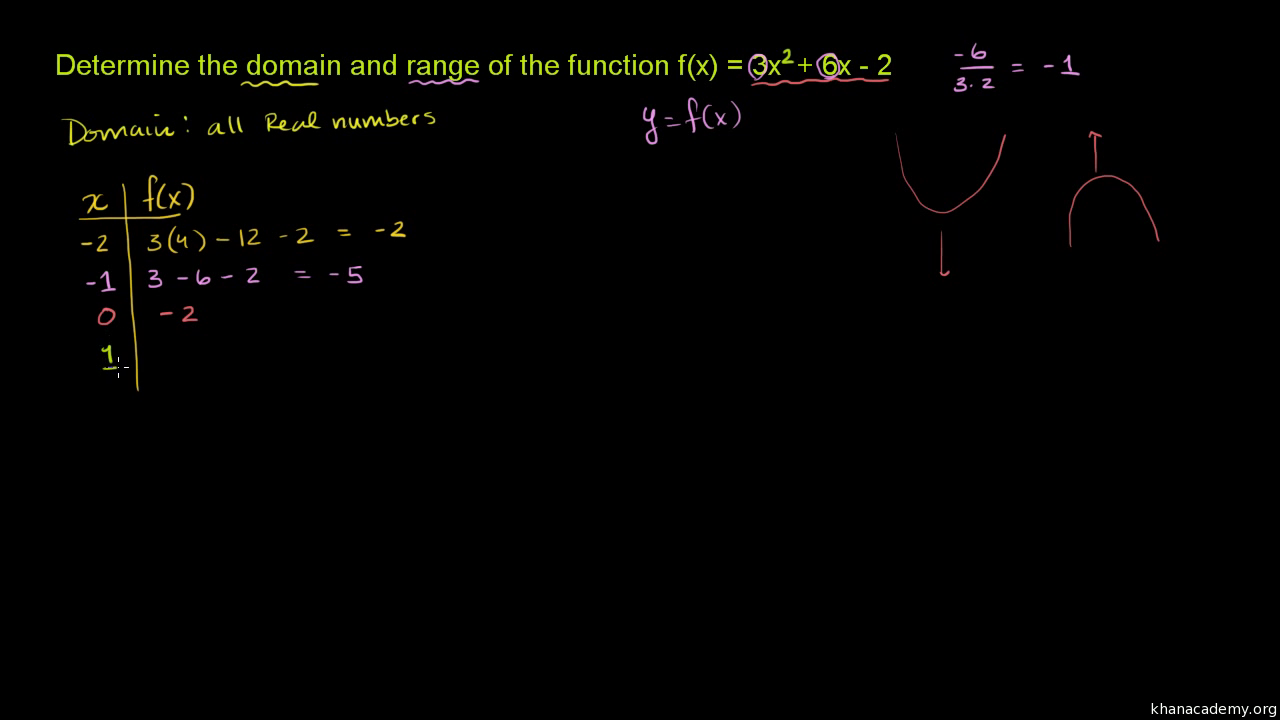



Domain And Range Of Quadratic Functions Video Khan Academy
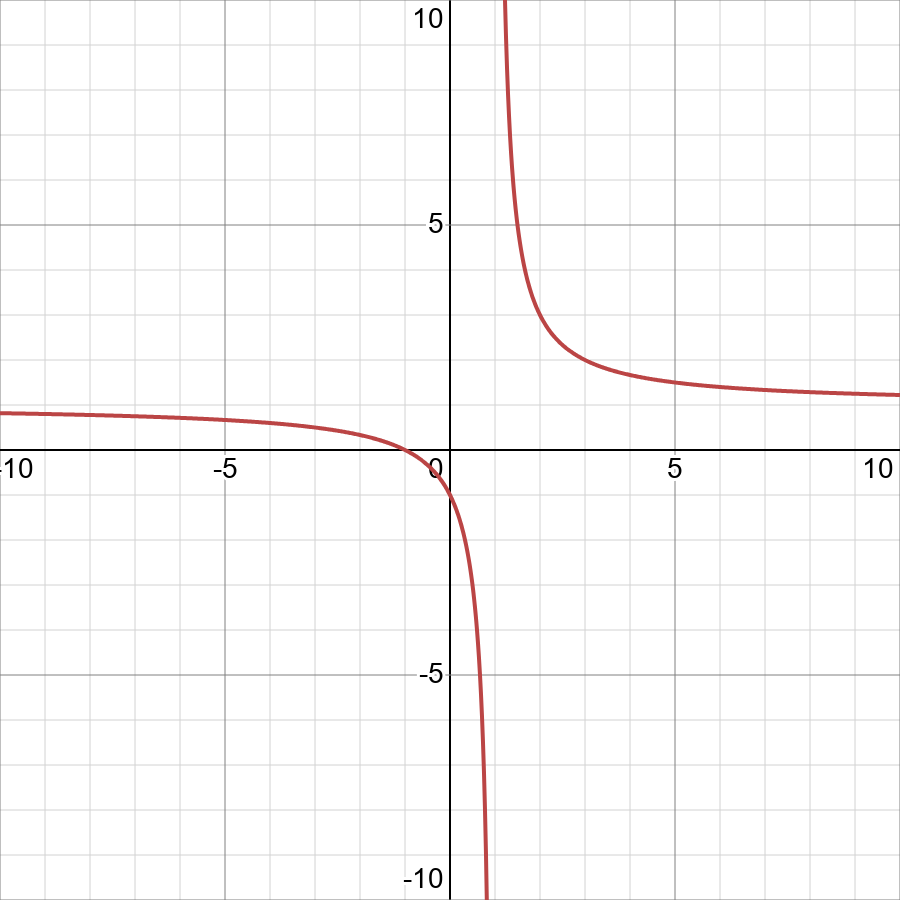
Explanation h(x) = 1 x 1 Domain Denominator must not be 0 ∴ x 1 ≠ 0 or x ≠ − 1 Domain is any real value except −1 So Domain is R,x ≠ − 1 Range is any real value except 0 So Rage is R,h(x) ≠ 0 graph {1/ (x1) 10, 10, 5, 5} Ans Answer link All these are real values Here value of domain (x) can be any real number Hence, Domain = R (All real numbers) We note that that Range f (x) is 0 or negative numbers, Hence, Range = (−∞, 0 Ex 23, 2 Find the domain and range of the following real function (ii) f (x) = √ ( (9 −x^2)) It is given that the function is a real functionCalculus Find the Domain and Range f (x)=1/ (x7) f (x) = 1 x − 7 f ( x) = 1 x 7 Set the denominator in 1 x−7 1 x 7 equal to 0 0 to find where the expression is undefined x−7 = 0 x 7 = 0 Add 7 7 to both sides of the equation x = 7 x = 7 The domain is all values of x x that make the expression defined Interval Notation
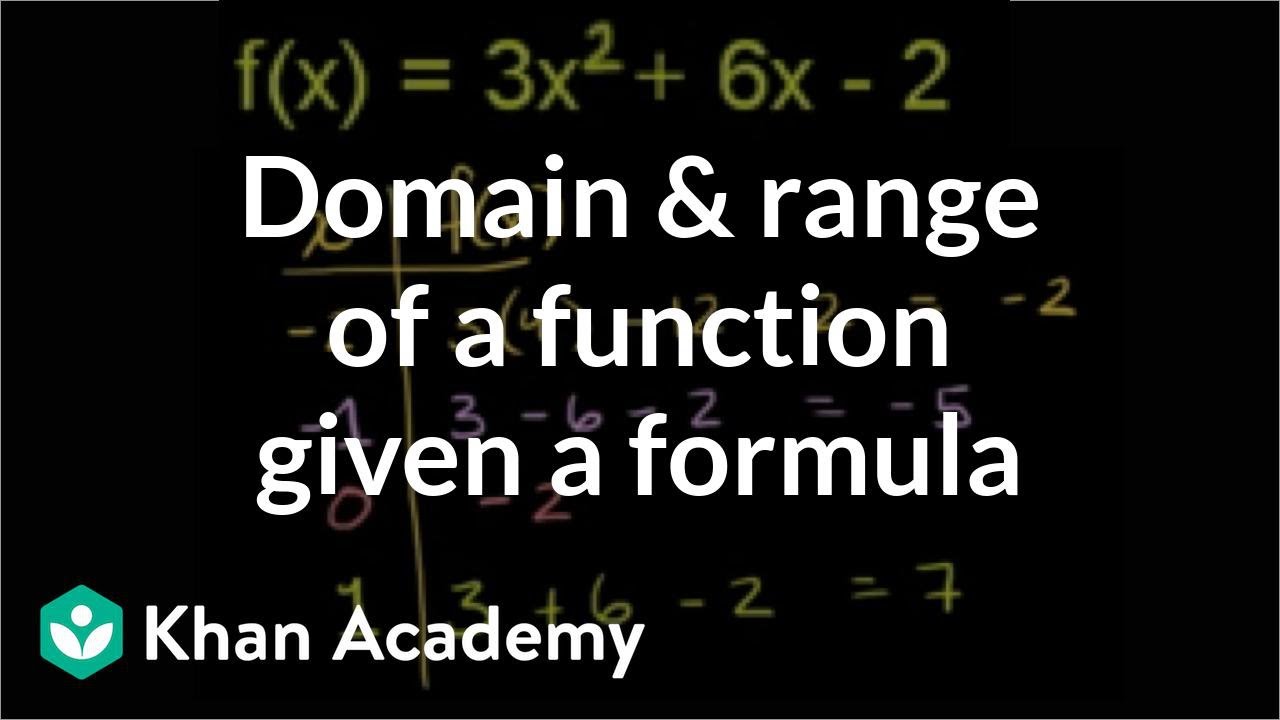



Domain And Range Of Quadratic Functions Video Khan Academy




A Draw The Graph Of F X X 1 Also Find The Domain And Range Of Maths Relations And Functions Meritnation Com
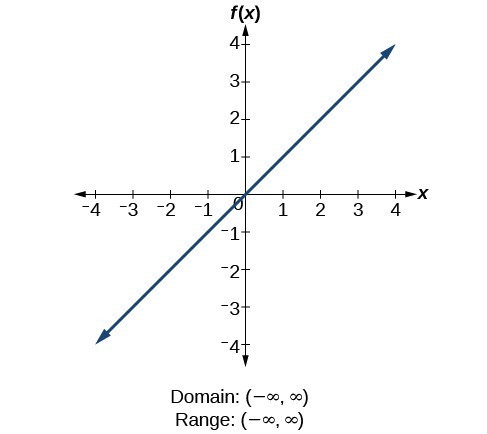
Given f(x) = 1/𝑥 , x ∈ R – {0} Finding f(x) at different values of x f(−2) = 1/(−2) = – 05 f(−15) = 1/(−15) = −10/15 = −2/3 = – 066 f(−1) = 1/(−1) = −1 f(−05) = 1/(−05) = −10/5 = – 2 f(025) = 1/025 = 100/25 = 4 f(05) = 1/05 = 10/5 = 2 f(1) = 1/1 = 1 fAnswer The domain is all real numbers, and the range is all real numbers f(x) such that f(x) ≤ 4 You can check that the vertex is indeed at (1, 4) Since a quadratic function has two mirror image halves, the line of reflection has to be in the middle of two points with the same yvalueQuestion 1 Find the domain and range of the following functions f(x) = x 3 Solution Domain A set of all defined values of x is known as domain Range The out comes or values that we get for y is known as range Domain for given function f(x) = x 3 For any real values of x, f(x) will give defined values Hence the domain is R



What Is The Range Of F X X 1 X 1 Quora




Find Domain And Range F X X 1 X 1 Maths Relations And Functions Meritnation Com
Find the Domain and Range f (x)=1 f (x) = 1 f ( x) = 1 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval Notation (−∞,∞) ( ∞, ∞) Set Builder Notation {xx ∈ R} { x x114 Range of a function For a function f X → Y the range of f is the set of yvalues such that y = f(x) for some x in X This corresponds to the set of yvalues when we describe a function as a set of ordered pairs (x,y) The function y = √ x has range; For instance, f(x) = itex\frac{1}{x3}/itex The domain is simply the denominator set equal to 0, {xl x≠3} However, range is found by solving for (isolating x to one side) and setting the denominator equal to zero x = itex3\frac{1}{y}/itex So range is {xl x≠0} This is a systematic method that I assume is the only way to find the range




Find The Domain And Range Of Each Of The Following Functions F X X 2 X 1 X 2 4x 3



Answer In Algebra For Dani Wagas
Rational functions f(x) = 1/x have a domain of x ≠ 0 and a range of x ≠ 0 If you have a more complicated form, like f(x) = 1 / (x – 5), you can find the domain and range with the inverse function or a graph See Rational functions Sine functions and cosine functions have a domain of all real numbers and a range of 1 ≤ y ≤ 1How to Find the Domain and Range of f(x, y) = ln(xy 2)If you enjoyed this video please consider liking, sharing, and subscribingYou can also help supportThe "" means "such that," the symbol ∈ means "element of," and "ℝ" means "all real numbers" Putting it all together, this statement can be read as "the domain is the set of all x such that x is an element of all real numbers" The range of f (x) = x2 in set notation is R {y y ≥ 0} R indicates range




Domain And Range Calculator Wolfram Alpha



3
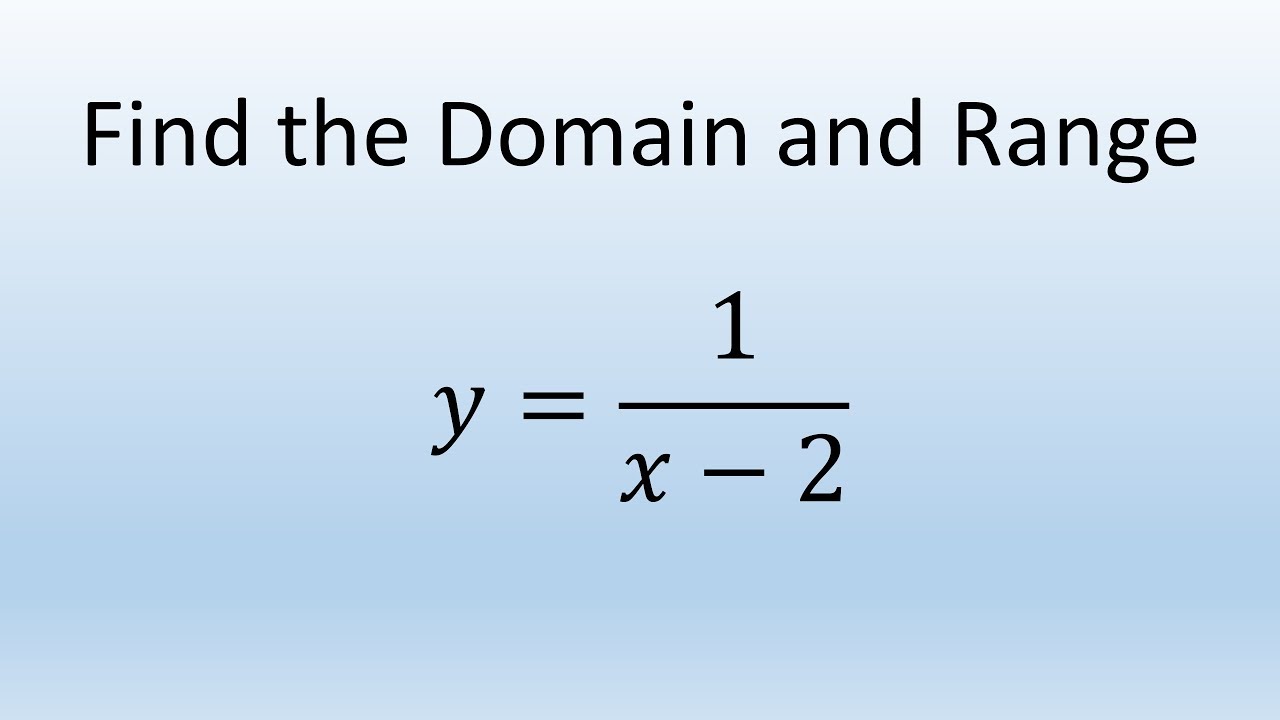
Arithmetic Mean Geometric Mean Quadratic Mean Median Mode Order Minimum Maximum Probability MidRange Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution f(x)=\frac{1}{x^2} domain\y=\frac{x}{x^26x8} domain\f(x)=\sqrt{x3} domain\f(x)=\cos(2x5) domain\f(xWhat are the domain and range of f (x) = (1/6)^x 2?Algebra Find the Domain and Range f (x)=1/ (x1) f (x) = 1 x − 1 f ( x) = 1 x 1 Set the denominator in 1 x−1 1 x 1 equal to 0 0 to find where the expression is undefined x−1 = 0 x 1 = 0 Add 1 1 to both sides of the equation x = 1 x = 1



For The Function F X X 2 5 Find The Domain And Range Enotes Com



3
Find the domain and range of the real function f (x) = x/1x^2 ━━━━━━━━━━━━━━━━━━━━━━━━━ ️Given real function is f (x) = x/1x^2 ️1 x^2 ≠ 0 ️x^2 ≠ 1 ️Domain x ∈ RSolution We have, f(x) = 1 1 − x2 Clearly, f(x) is defined for all x ∈ R except for which x2 − 1 = 0 ie, x = ± 1 Hence, Domain of f = R − { − 1, 1} Let f(x) = y Then, 1 1 − x2 = y ⇒ 1 − x2 = 1 y ⇒ x2 = 1 − 1 y = y − 1 yNEW Interested in Finding Out the Top "{{3}} Challenges that Can Get YOU in Trouble with Math"?




Find The Domain And The Range Of The Real Function Defined By F X Sqrt X 1



Graph The Function F X Ln X 1 Determine The Domain Range And Vertical Asymptote Enotes Com
The terms within the radical are x 3 Find domain and range of f (x)= square root of (x 1) (3 x) Enter your answer for the domain using interval notation Set the radicand in greater than or equal to to find where the expression is defined Fx x 2 2 x 1 Solution to Example 3Figure 3 Domain and range of a function and its inverse When a function has no inverse function, it is possible to create a new function where that new function on a limited domain does have an inverse function For example, the inverse of f ( x) = x \displaystyle f\left (x\right)=\sqrt {x} f (x What is the domain and range of this function?



Domain And Range Examples Domain And Range Of Functions




Find Domain And Range Of Real Functions 1 F X X 2 3 X 2 F X 1 Sqrt X 5 3 F X X 1 X 2
Read the book Dr Pan just finished!!Grab a copy here h For example, the function \(f(x)=\dfrac{1}{\sqrt{x}}\) has the set of all positive real numbers as its domain but the set of all negative real numbers as its range As a more extreme example, a function's inputs and outputs can be completely different categories (for example, names of weekdays as inputs and numbers as outputs, as on anFind the domain and range of the function f (x) = 2 − sin3x1 Solution Solution f (x) = 2 − sin3x1 Here, 2 − sin3x can never be zero as sin3x will always less than 2 ∴ Domain of f (x) will be x ∈ R Now, f (x) will be maximum when 2 − sin3x is minimum 2 − sin3x will be minimum when sin3x = 1



Find The Domain And The Range Of The Real Function F Defined By F X Sqrt X 1 Sarthaks Econnect Largest Online Education Community
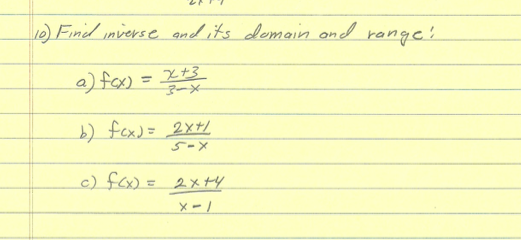



Find Inverse And Its Domain And Range F X X Chegg Com
Given f(x) = √x 1 To find the domain and range of function Explanation So the domain of a function consists of all the first elements of all the ordered pairs, ie, x, so we have to find the values of x to get the required domain Given, f(x) = √x 1 Now for real value of x1≥0 ⇒ x≥1 Hence the domain of f = 1, ∞) And the range of a function consists of all the second
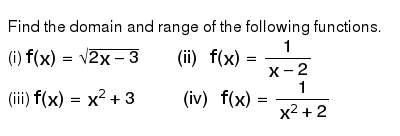



Find The Domain And Range Of The Following Functions I F X Sqrt 2x 3 Ii F X 1 X 2 Iii F X X 2 3 Iv F X 1 X 2 2
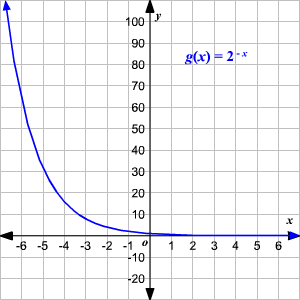



Domain And Range Of Exponential And Logarithmic Functions



Solution Sketch F X X 1 If X Lt 1 0 If 1 Amp 04 X Amp 04 1 X 1 If X Gt 1 Find The Domain And Range Using Interval Notation



What Is The Domain And Range Of F X X 1 X 2 1 X 3 Quora



1
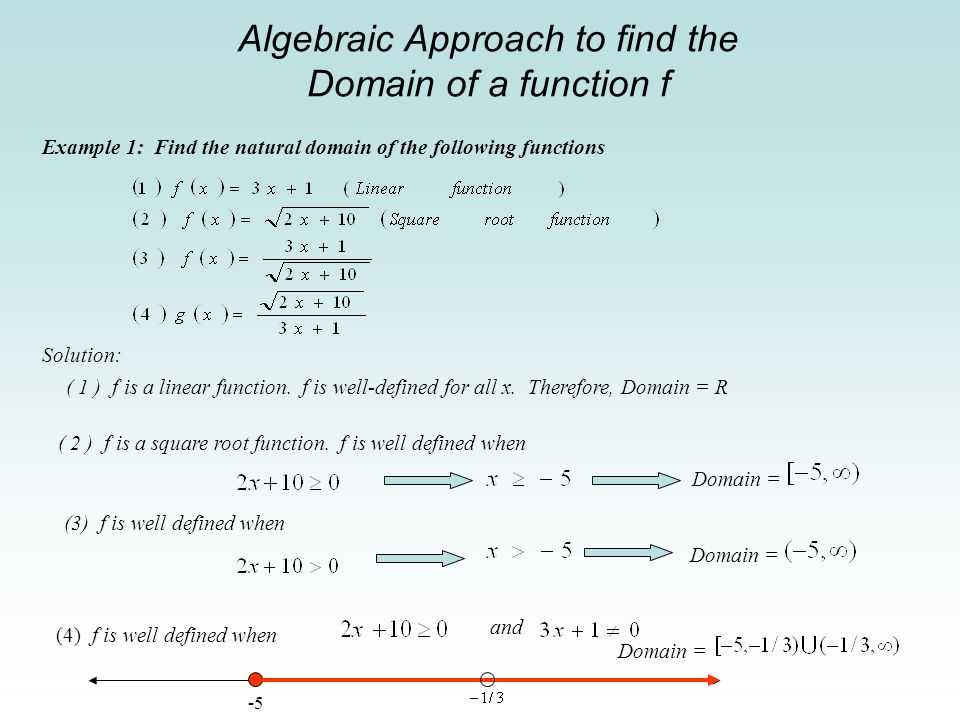



Interpretation Of Domain And Range Of A Function F Ppt Download




Find Domain And Range Of Real Functions 1 F X X 2 3 X 2 F X 1 Sqrt X 5 Youtube
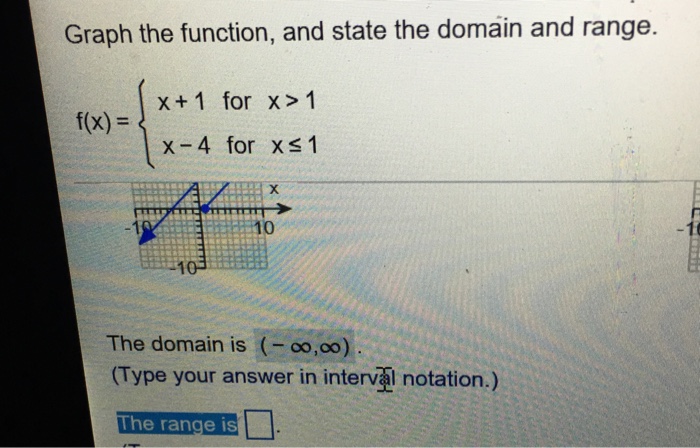



Graph The Function And State The Domain Range F X Chegg Com
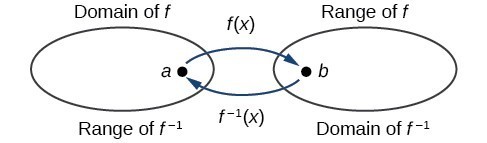



Determine The Domain And Range Of An Inverse Function College Algebra
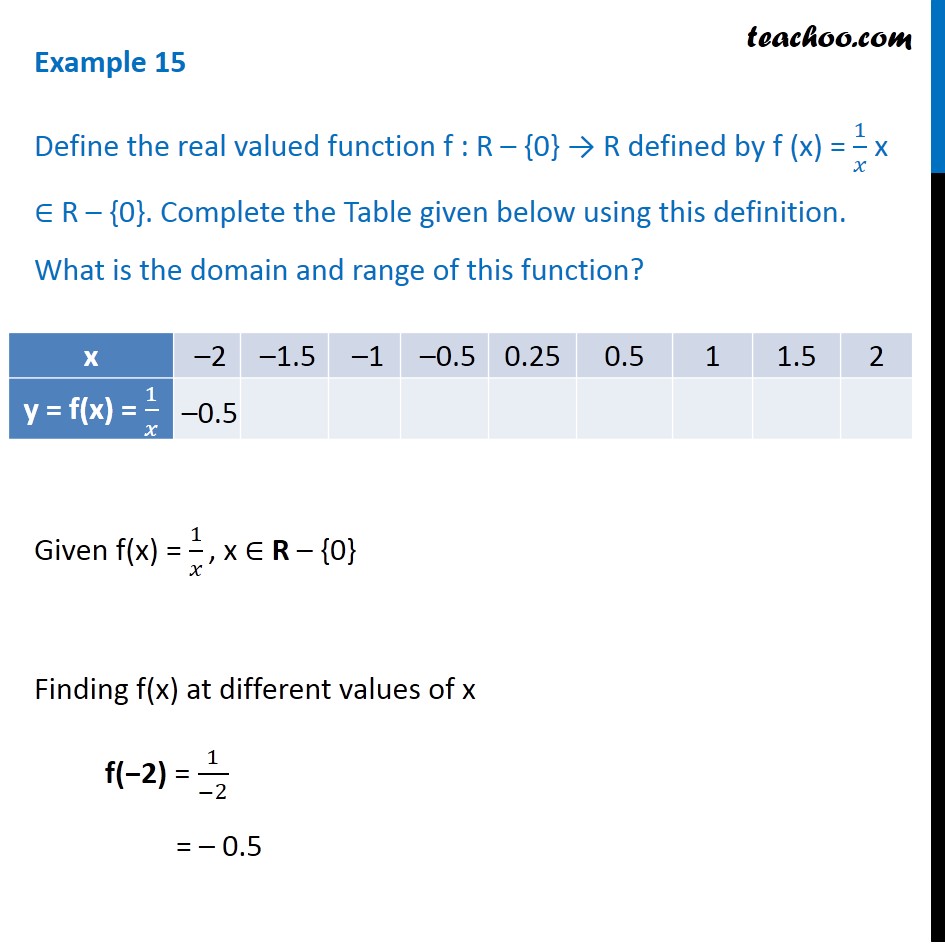



Example 15 F X 1 X What Is The Domain And Range Examples




Find The Domain And Range Of The Following Functions I F X X 2 Ii F X X 1 3 X



Find The Domain And Range Of F X X 1 Youtube



Introduction To Domain And Range Relations And Functions Geeksforgeeks
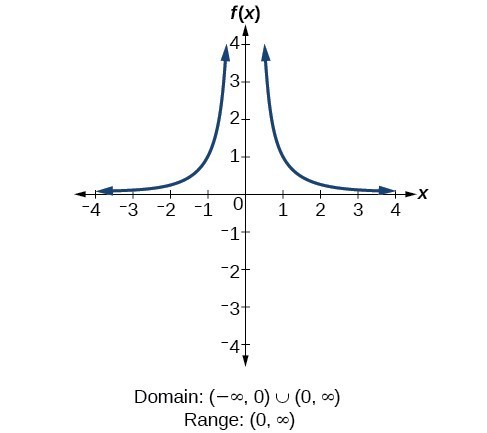



Find Domains And Ranges Of The Toolkit Functions College Algebra




Find The Domain And Range Of The Function F X 2 X 5
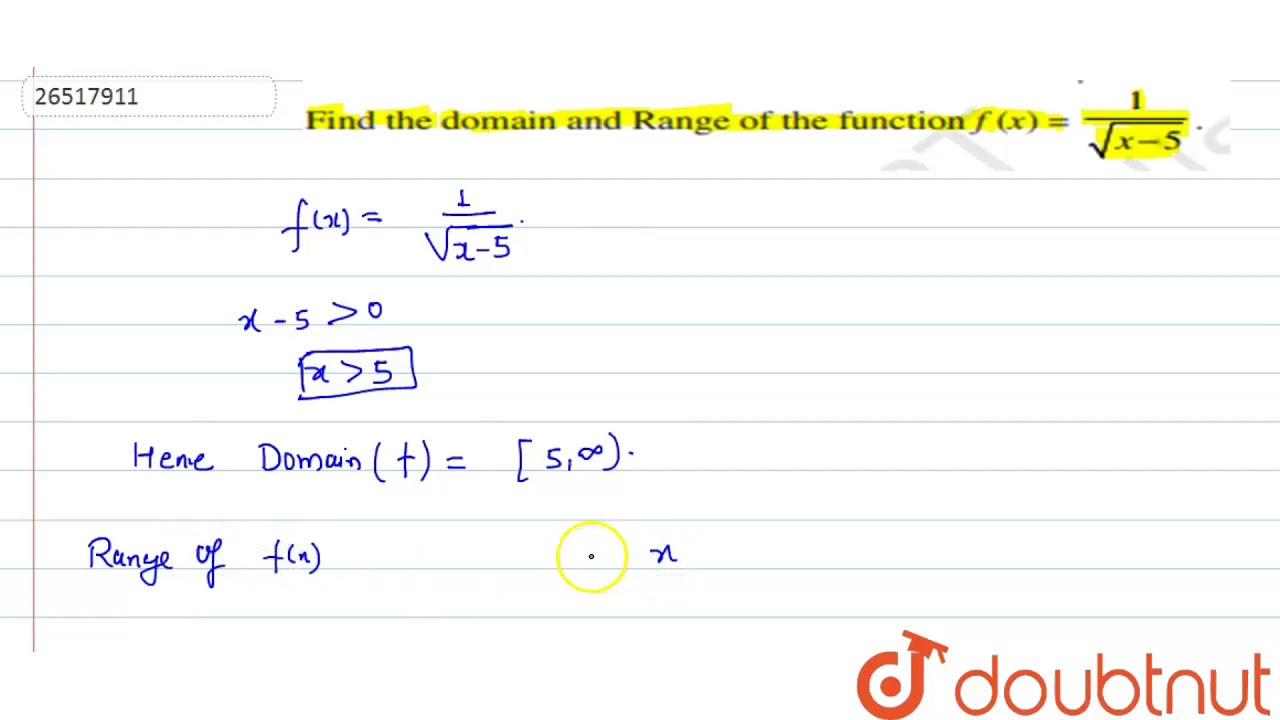



Find The Domain And Range Of The Function F X 1 Sqrt X 5 Youtube
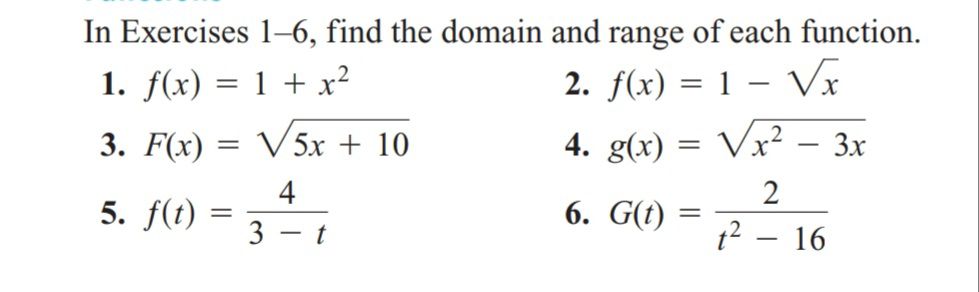



Answered In Exercises 1 6 Find The Domain And Bartleby
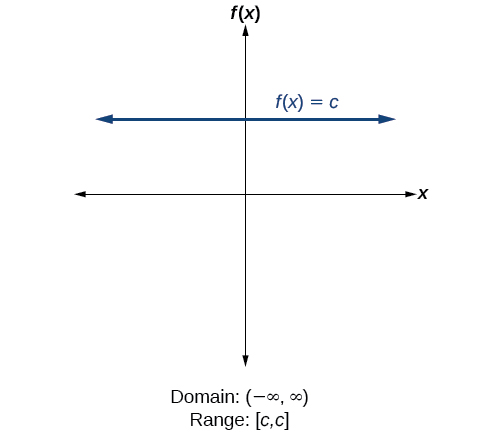



3 3 Domain And Range Mathematics Libretexts




Domain And Range Free Math Help




Find Domains And Ranges Of The Toolkit Functions Math 1314 College Algebra
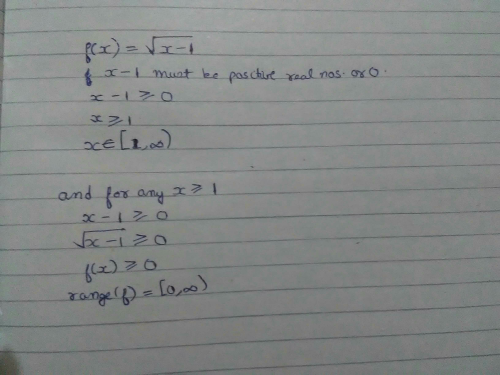



Find The Domain And Range Of Real Function F Defined By F X Underoot Of X 1 Edurev Class 11 Question



Misc 4 Find Domain And Range Of F X Root X 1 Chapter 2



Www Math Colostate Edu Clayton Teaching M113f09 Homework Hw1solutions Pdf



Misc 5 Find Domain And Range Of F X X 1 Class 11



What Is The Domain And Range Of Y 1 X Quora



Find The Domain And Range F X X 1 X 1 Displaystyle Fleft Xright Frac X 1 X 1 Snapsolve



What Is The Domain And Range For The Following Function And Its Inverse F X 3x 1 2 Mathskey Com
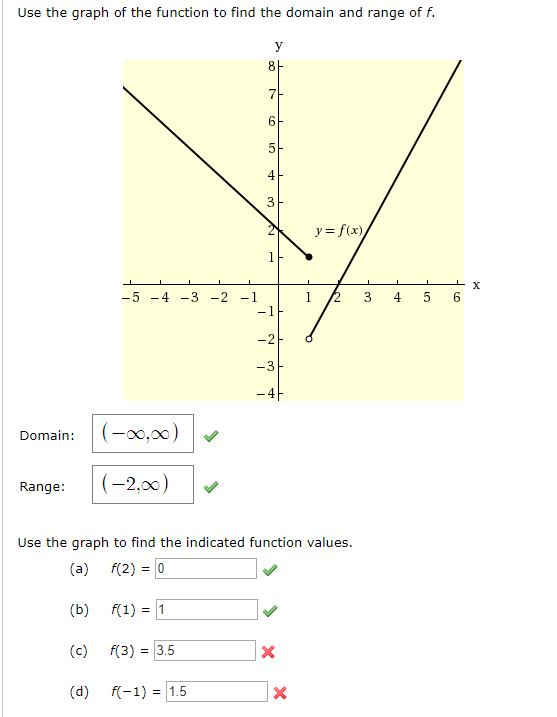



Use The Graph Of The Function To Find The Domain And Chegg Com
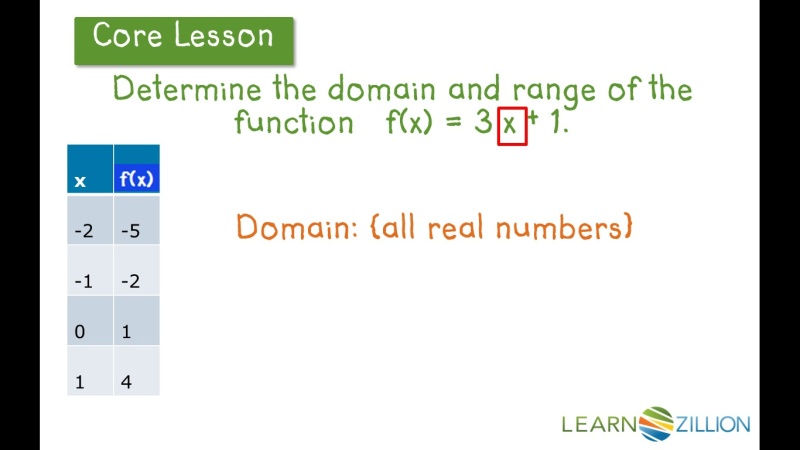



Find The Domain And Range Of A Function Learnzillion
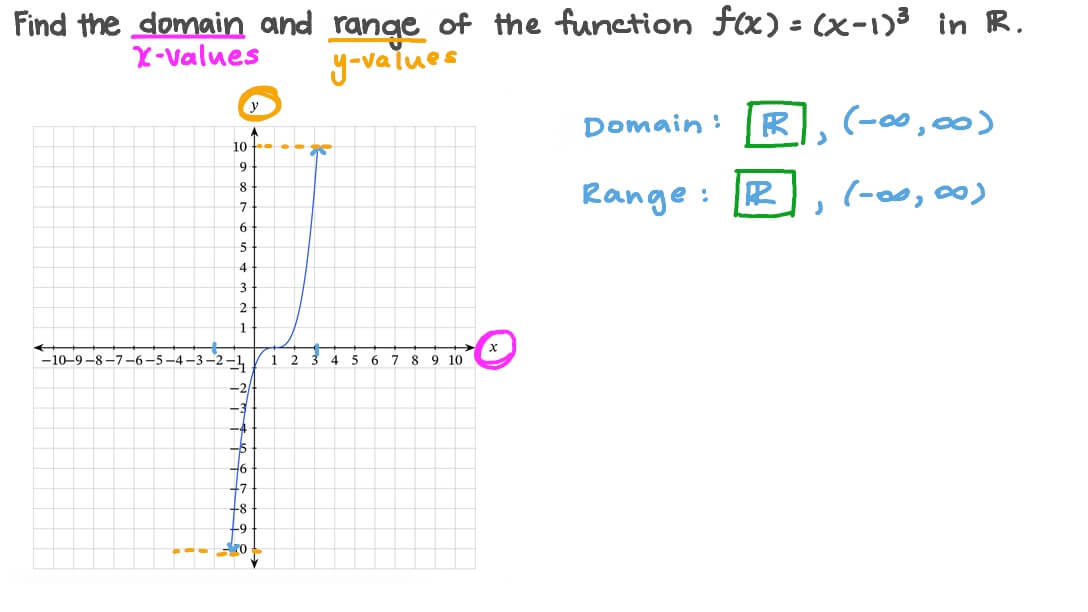



Question Video Finding The Domain And Range Of A Cubic Function Given Its Graph Nagwa



Solved In Exercises 1 6 Find The Domain And Range Of Each Function F X 1 X 2




Section 1 1 Relations And Functions Relation Domain Range Function Example 1 State The Domain And Range Of Each Relation Then State Whether Ppt Download



Domain And Range Of Rational Functions



Http Fsw01 c Cuny Edu Luis Fernandez01 Web Teaching Classes Math30 Nikos 30sp10 Funex Pdf
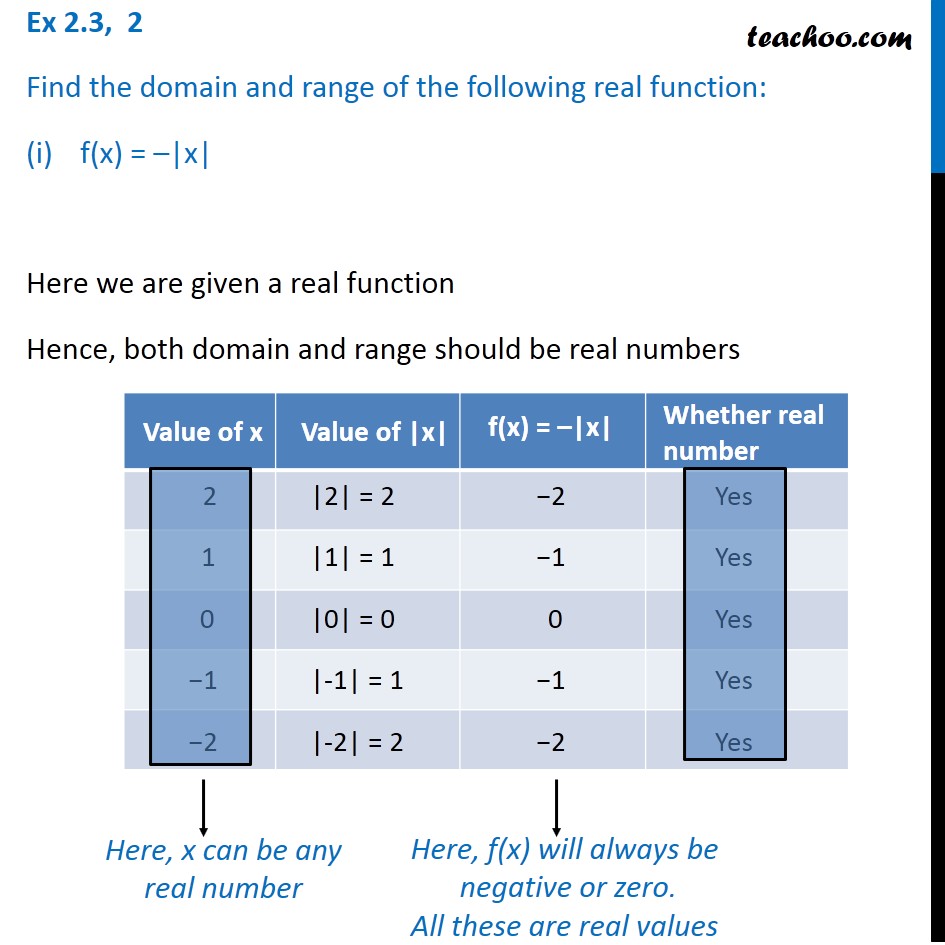



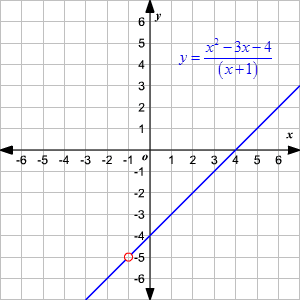
Ex 2 3 2 Find The Domain And Range Of The Function F X X




Find The Domain And Range Of The Function F X 1 1 X 2 X In R X 1 Dot Youtube



Domain And Range Of Rational Functions




Find Domain And Range Of F X X 1 C 2x 8 Maths Relations And Functions Meritnation Com



Solution Find The Domain An Range Of F X X 1 X




The Domain Of The Function F X 1 1 E 1x 1 Is



Find Domain And Range Of Function Y 1 X 2 Youtube



The Domain And Range Of The Function F X X 2 Is Donimain




Domain And Range Of 1 X 2 2x 8 Youtube
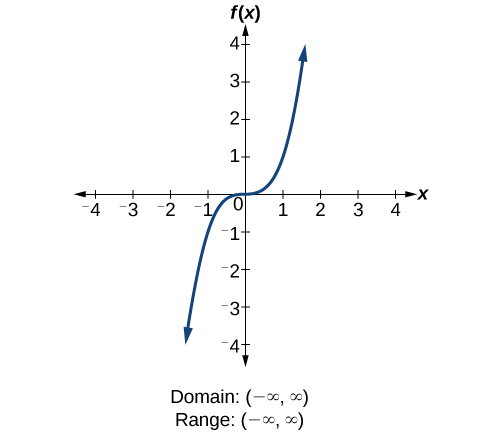



3 3 Domain And Range Mathematics Libretexts




Domain And Range Of Functions
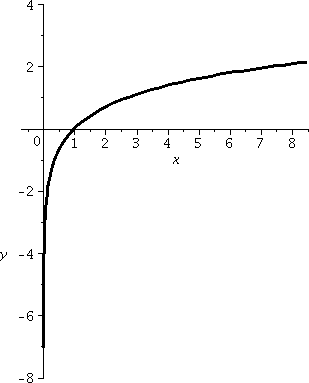



Domain And Range And Composition Of Functions
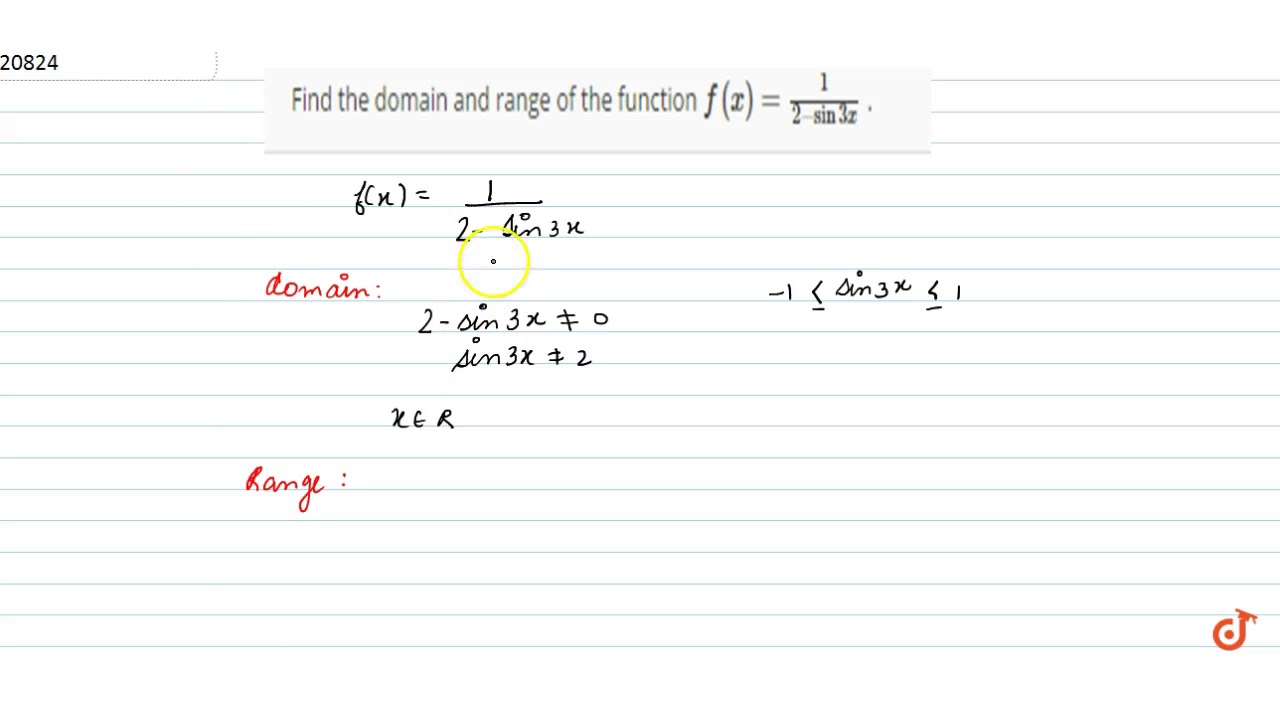



Find The Domain And Range Of The Function F X 1 2 Sin3x Youtube




Find The Domain Or Range Of Following Function Tex F X Sqrt X 1 3 X Tex Brainly In




What Are The Domain And Range Of F X 1 5 X Brainly Com




Find The Domain And Range Of The Function F X X 1 X 2 Brainly In
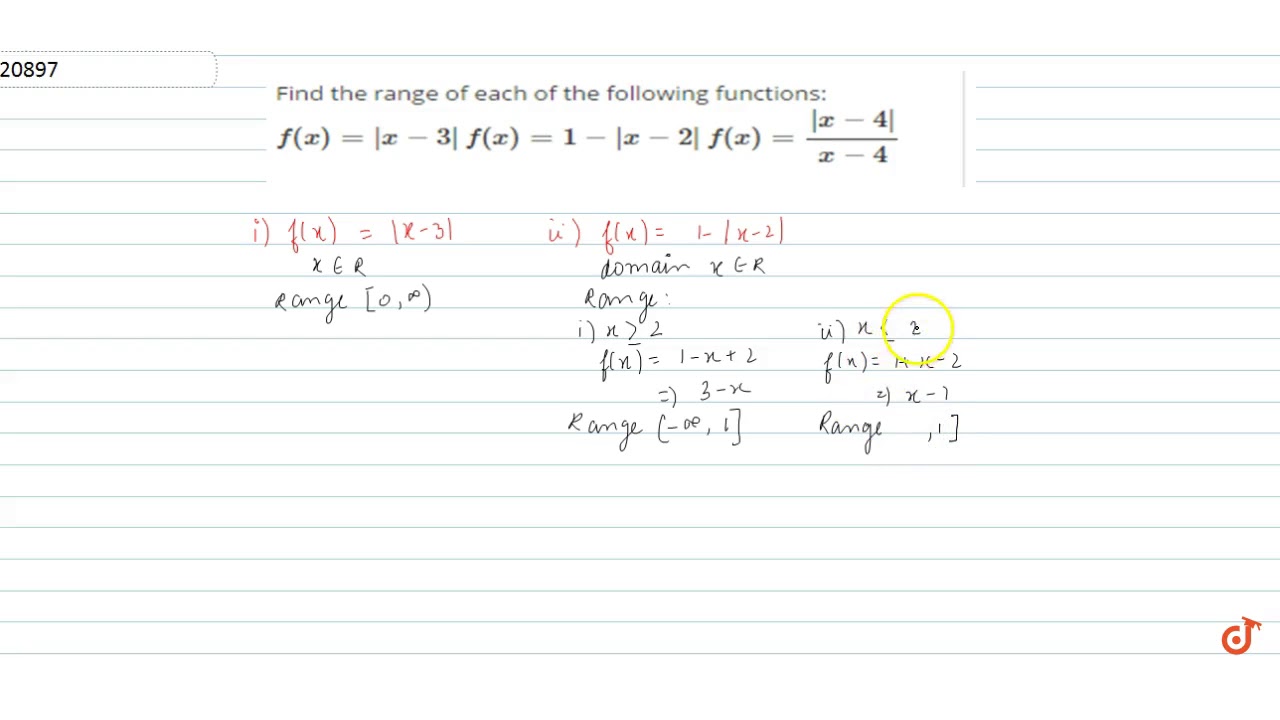



Find The Range Of Each Of The Following Functions F X X 3 F X 1 X 2 F X X 4 Youtube
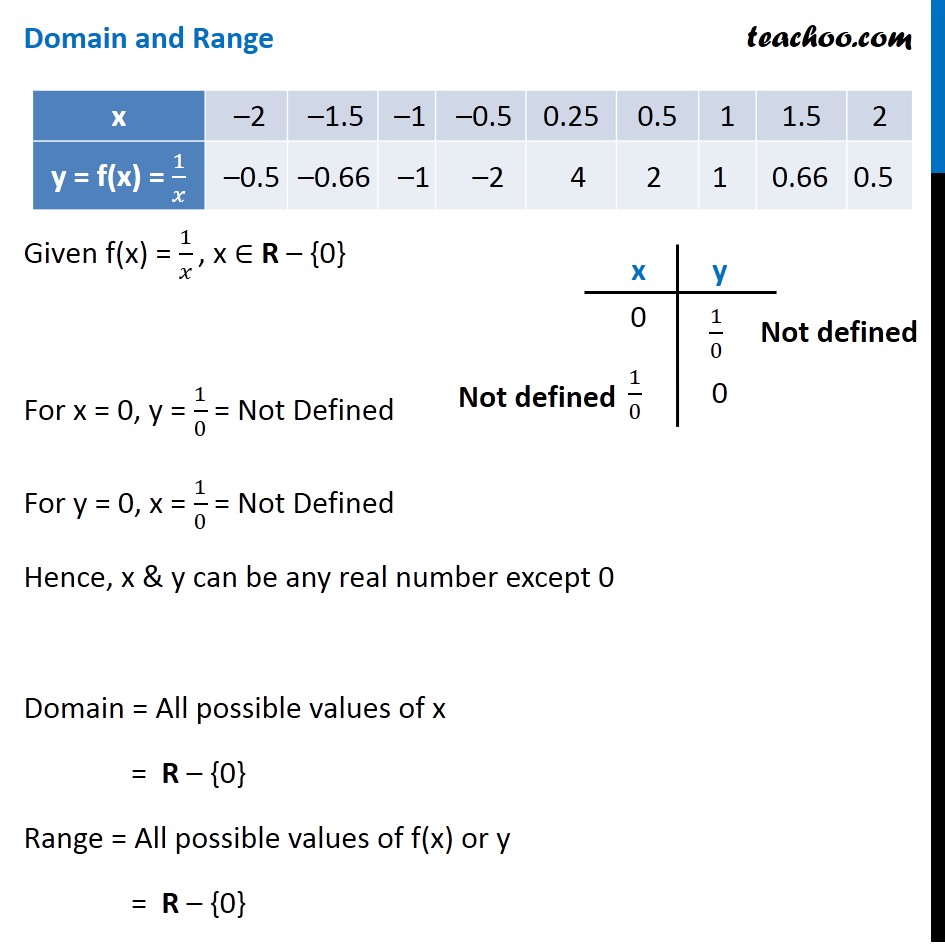



Example 15 F X 1 X What Is The Domain And Range Examples
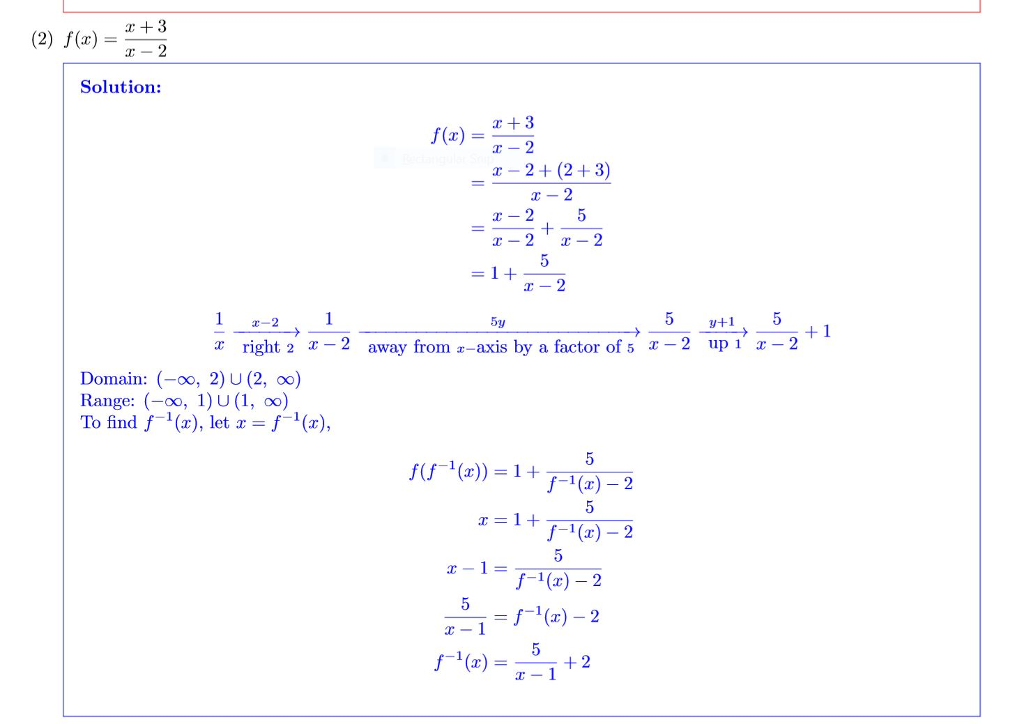



The Problem Was To Graph The Function Find The Chegg Com




Answered 6 Determine The Domain And Range Of Bartleby



What Is The Range Of The Function F X X 1 Quora




Find The Domain And Range Of The Function F X 1 1 X 2 X In R X 1 Dot
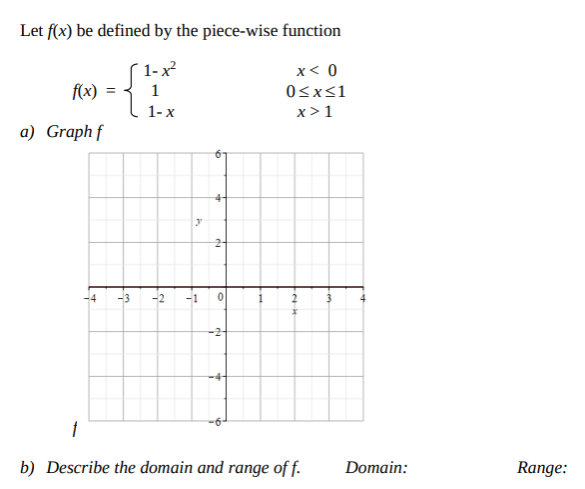



Answered Let F X Be Defined By The Piece Wise Bartleby




How To Find The Domain And Range Of A Function 14 Steps
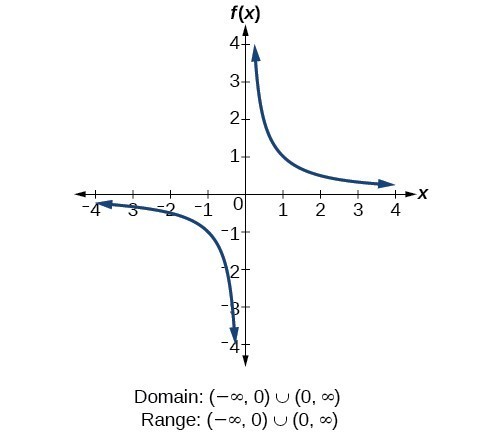



Find Domains And Ranges Of The Toolkit Functions College Algebra
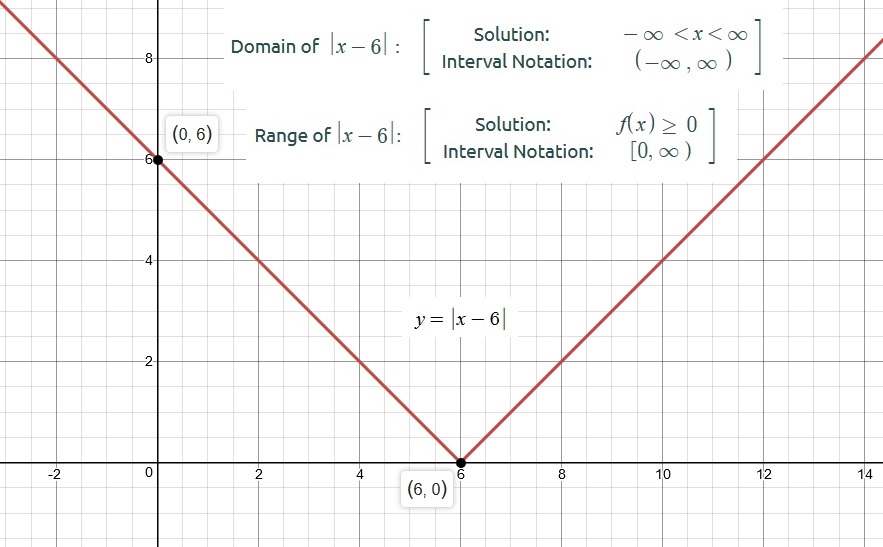



How Do You Find The Domain And Range Of Y X 6 Socratic




Let F X Ln X Y 1 Find And Sketch The Domain Of F What Is The Range Of F Study Com
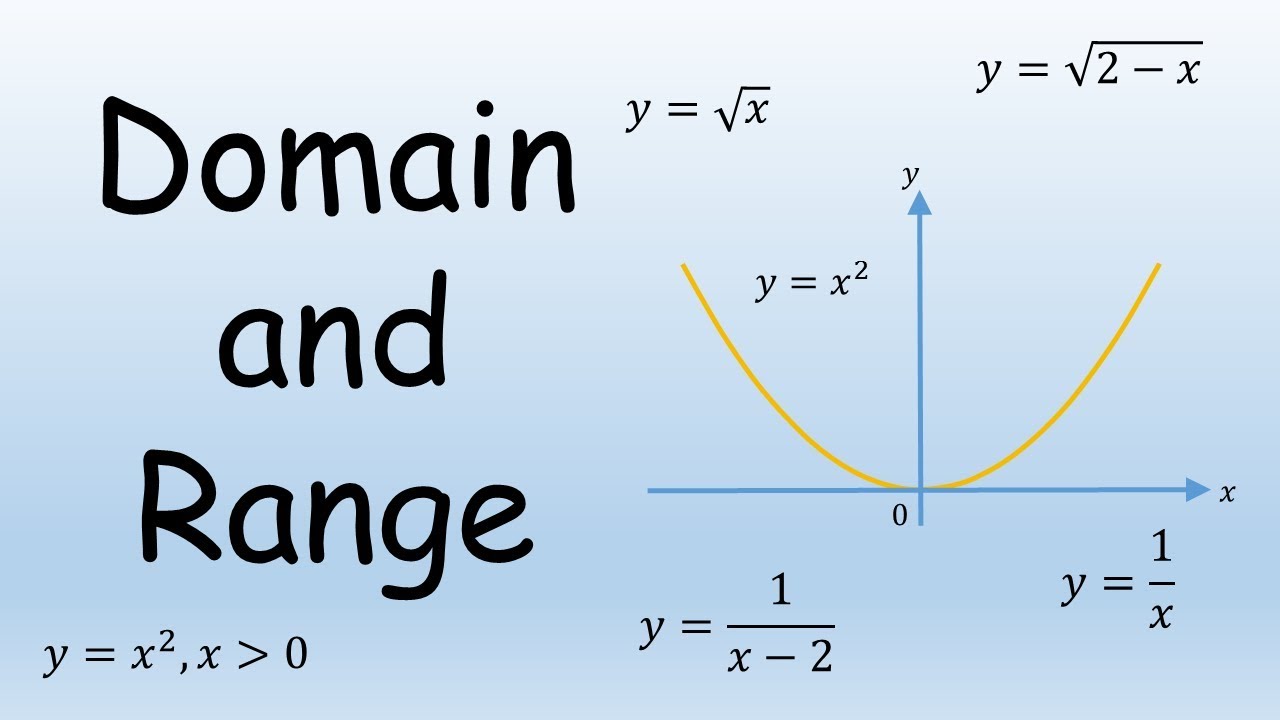



Find Domain And Range Of Function Y 1 X 2 Youtube
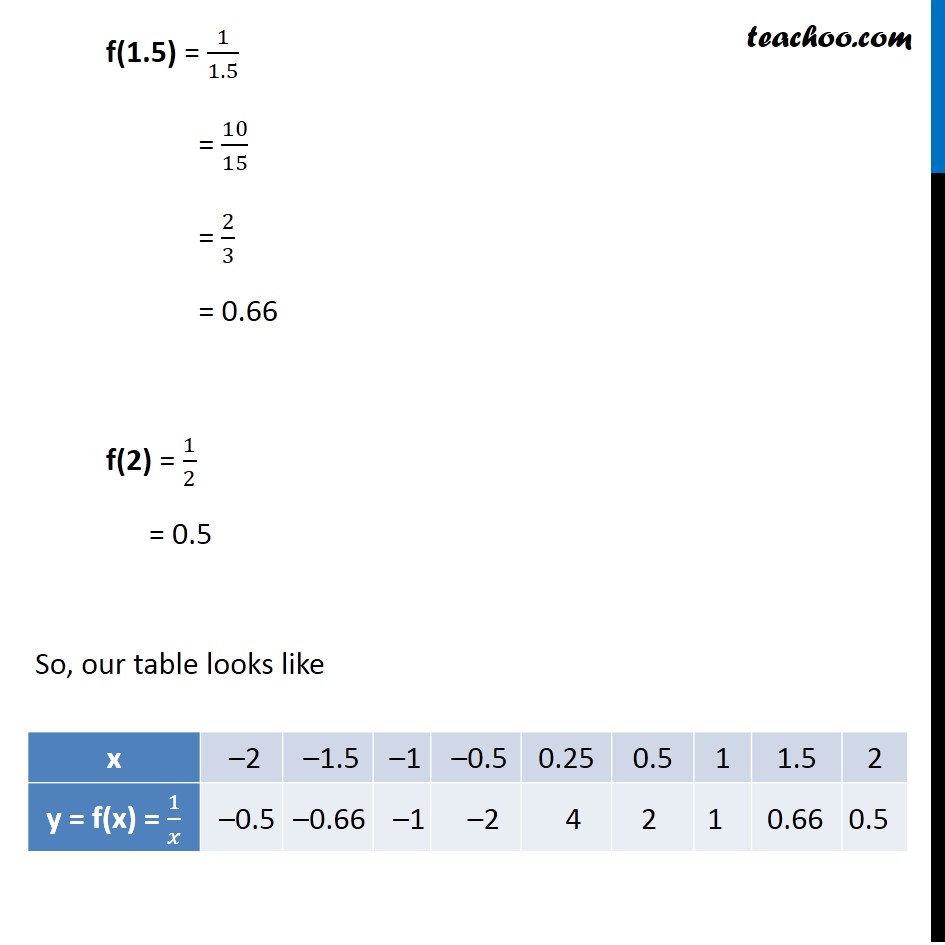



Example 15 F X 1 X What Is The Domain And Range Examples




Find The Domain And The Range Of The Real Function F X 1 X 3




Section 1 5 Combinations Of Functions Ppt Download



Domain Range A Function



Domain And Range Of A Function



Search Q Domain And Range Table Tbm Isch
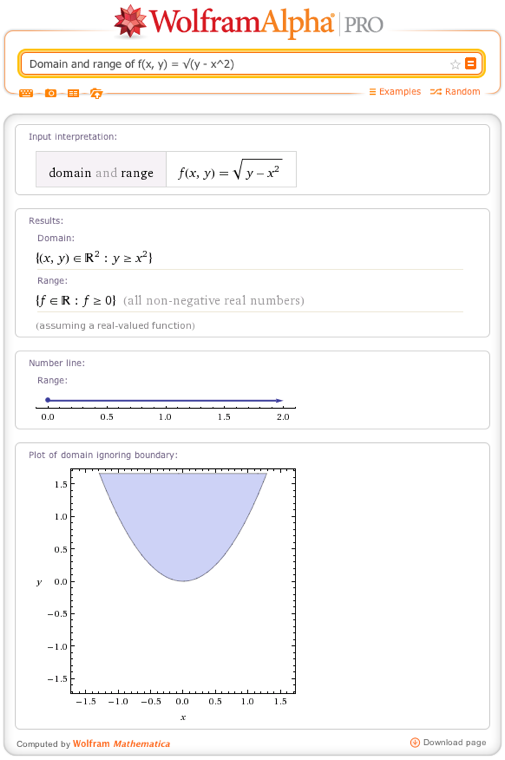



Solve Domain And Range Of A Function With Wolfram Alpha Wolfram Alpha Blog
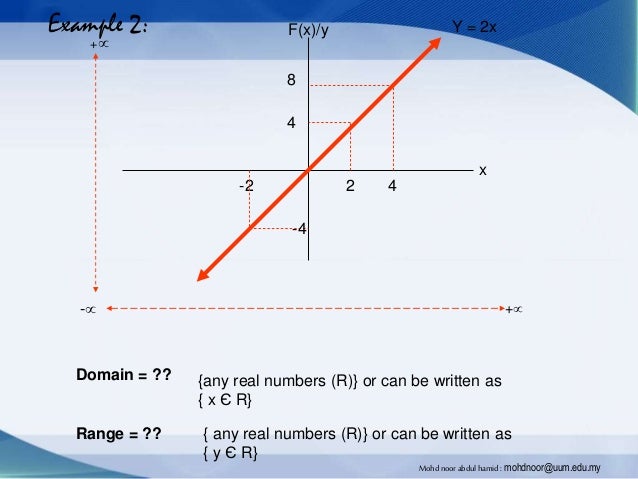



Introduction To Function Domain And Range Mohd Noor




How To Find Domain And Range Of The Inverse Of A Relation Sat Math




How Do You Graph F X 2 X 1 3 And State The Domain And Range Socratic
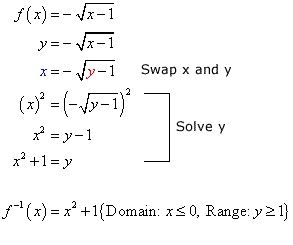



Inverse Of Square Root Function Chilimath
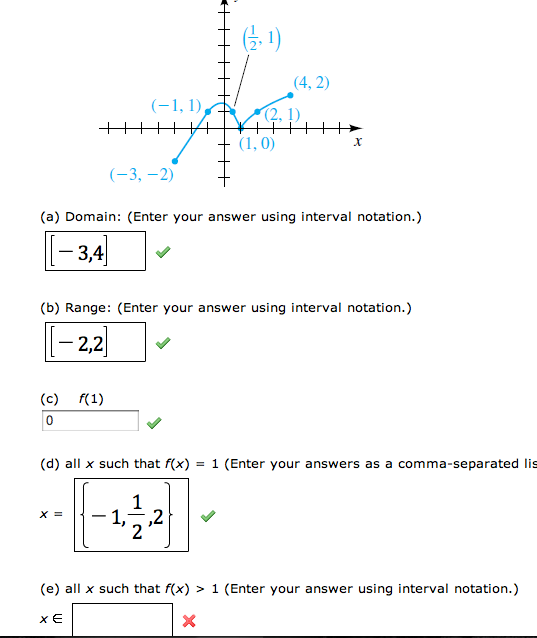



Domain Range F 1 All X Such That F X 1 X Chegg Com




What Is The Domain And Range Of F X Abs X 3 5 2 Socratic




What Are The Domain And Range Of F X 1 6 X 2 Brainly Com
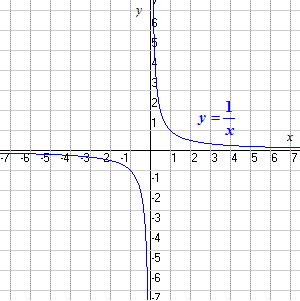



Domain And Range




Domain And Range Of F X X 3 X 3 Are Respectively
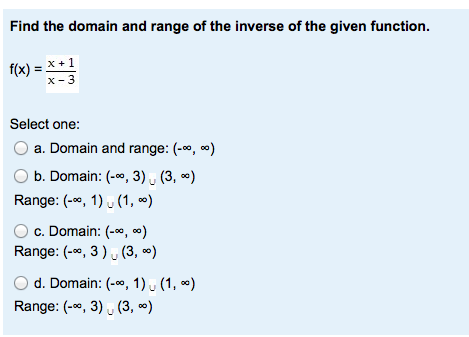



Find The Domain And Range Of The Inverse Of The Given Chegg Com




Domain And Range



0 件のコメント:
コメントを投稿